Why the Properties of Operations are the Key to Multiplication Fluency

"How can I help this child build fluency with their multiplication facts?" is a question I get asked all the time. Whether it's from parents or teachers, tutors or interventionists, this is such a common question.

There is no one right way to teach multiplication fact fluency. There is no magic key that will make that lightbulb click on. That's because 'tricks' in math usually don't hold a lot of ground over time. There is no longevity to math tricks. Yes, they might help a child build fluency in the short term, but will that child actually be able to apply these facts to more challenging problems? Will they build the flexibility in problem solving that is required to take on more challenging multiplication problems with larger numbers? Not necessarily.

However, if we start with a foundation of using strategies, and building conceptual understanding, rather than tricks or pneumonic devices, children will be able to apply their knowledge and understanding of multiplication facts to larger problems.

There are an abundance of multiplication strategies that children can use to build understanding of multiplication concepts (which leads to fluency). Many visual models that are hugely important for building this understanding. But today, I want to dive into an often forgotten set of 'strategies' that can unlock some keys to problem solving with multiplication for our children. These strategies are 'using the properties of operations to multiply.'

Here's a quick breakdown of the properties of multiplication and a brief explanation of how children can use them to multiply.

The commutative property: states that you can reverse the order of the factors and the product remains the same. For example 3 x 4 = 4 x 5 (which is to say that 3 x 4 is the same value as 4 x 3) because 12 = 12.

This property can be proven by showing

children that you can draw three groups with 4 dots in each group and then also draw 4 groups with 3 dots in each group, and in both models, there are 12 total dots. You can also use my favorite way to model this property which is to draw a 3 x 4 array. Then simply take the paper and rotate it to show that it is the same thing as a 4 x 3 array without changing anything (check out my latest reel on my Instagram for a model of this)! So why is it important? Because if a child is given a problem such as 8 x 4 and they're not confident in their 8s facts, but they do know their 4s facts then they can think of this problem as 4 x 8 and they might 'just know' the value. They also might be able to switch it around to more easily break it apart into let's say (4 x 4) + (4 x 4). I like to help children remember what the commutative property is by saying that 'I commute to work which means I move around to get to work--so you can move around the order of the factors and the product remains the same.' Although, it is not critical that they remember the name of the property, just that they know and understand that the property works.

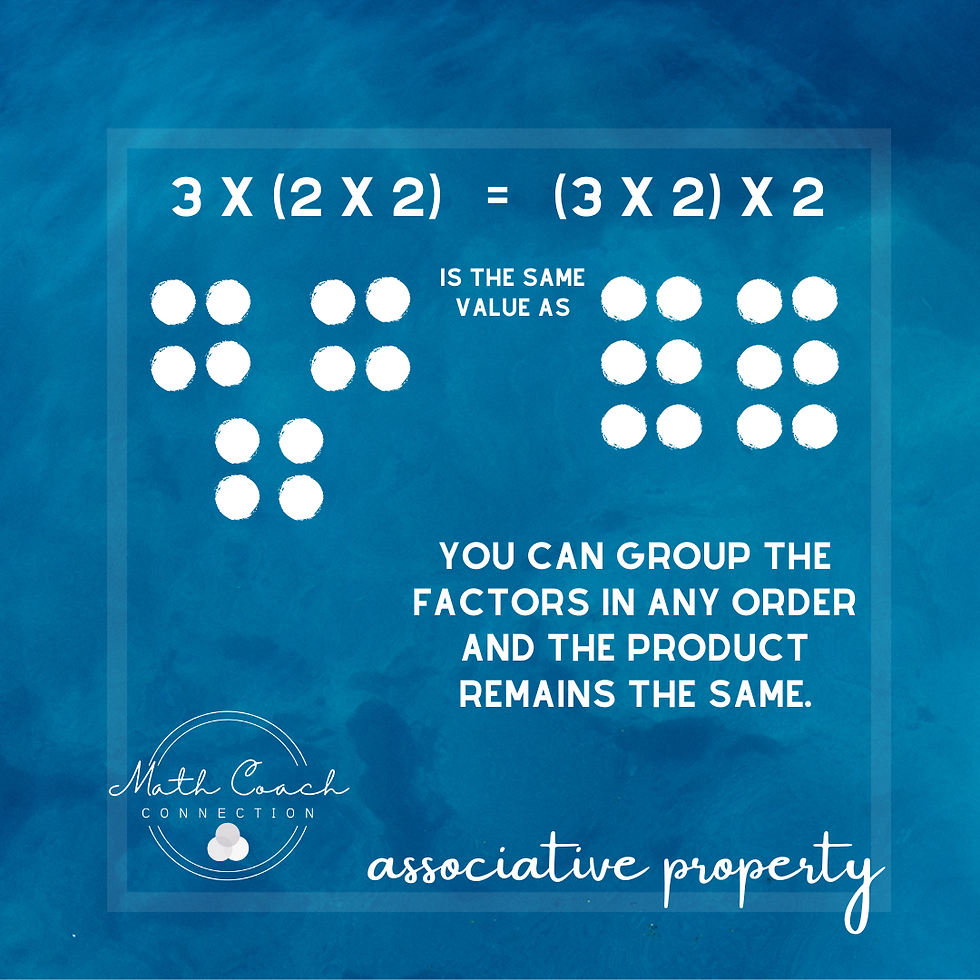
The associative property: states that you can group the factors in any order and the product remains the same. For example, if given the problem 5 x 4 x 2, a child can either find the value by multiplying (6 x 4) x 2 or by multiplying 6 x (4 x 2). Why is this an important strategy? It is especially helpful with math story problems, and it makes some of these more challenging, larger problems more accessible to children so that they can use multiplication facts they know to help them access more challenging problems. In the first example (6 x 4) x 3, a child would first multiply 6 x 4 to get 24. Then they would have to multiply 24 x 2. If a child is not confident with multiplying 2 digit numbers (over 12) yet, then this problem would provide a challenge for them. However, if they group the problem in a different way, looking at it as 6 x (4 x 2), then the problem becomes 6 x 8, which is likely a much more accessible problem to solve than 24 x 2 (especially at the 3rd grade level). I help children remember this property by saying that you can 'associate' yourself with any people you choose, so one day you might associate yourself or 'hang out with' Johnny, and the next day you might hang out with a new friend, Carlos, but no matter what, the three of you are all still friends. Kind of a lame way to describe it, but it helps children think about the meaning of the word 'associate.'

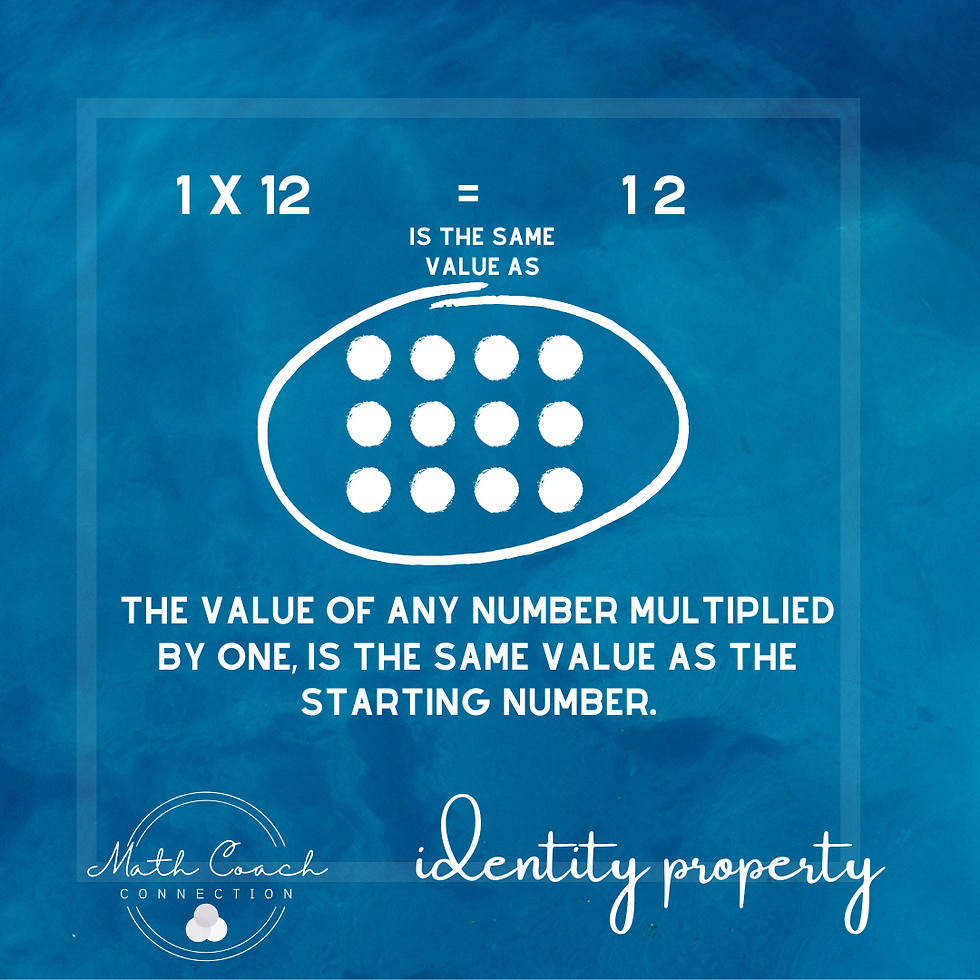
The identity property: there is nothing fancy about this property, but it states that any number multiplied by one, equals itself. Because one group of 5 is 5 and one group of 6 is 6 and one group of 7 is 7 and one group of any number is itself. This can (and should) be modelled to prove to students why it works, and is mainly helpful just for building a foundation of multiplication understanding. I tell my students that this one is like a number looking in a mirror--it sees itself. So when a number is multiplied by one, it keeps the same identity.

The distributive property: this is a BIG ONE. Arguably the most important property of operation for building understanding and fluency with larger multiplication problems. The distributive property states multiplying the sum of two (or more) addends by a number gives the same product as multiplying each addend by the number individually and then adding the products together.

Here's an example. If given the problem 8 x 12, you can break 12 apart into 10 + 2. Then multiply 8 x (10 + 2) which is (8 x 10) + (8 x 2), so 80 + 16 = 96. This is extremely helpful because it allows problem solvers to use smaller problems that they know, such as 8 x 10 or 8 x 2, to access a larger problem (8 x 12). It supports mental math strategies as children build fluency with those smaller problems because as they learn to break problems down in this way, they might be able to break it apart into smaller problems that they can solve mentally. This can be proven by using an array and open area model to show that a larger array can be broken apart into smaller arrays, that when put all together, equal the total value of the larger array. Why is this important? Just think of how helpful that would be with a large problem like 8 x 65 or 28 x 65 and so on. This lends itself to the partial products strategy which is a great gateway to transition from visual models, to more efficient symbolic models like the standard algorithm (but with enough conceptual understanding behind it to build the foundation before jumping to the algorithm). It helps children understand why this break apart strategy works so that they can apply it to larger problems. I help students remember this one by thinking about 'distributing' like dealing out a deck of cards. You have to 'deal' or 'distribute' the first factor across all the parts of the second factor (the addends you broke it apart into) to find the product. **And again, I want to emphasize that it is not critical that children know the names of the properties, but rather that they understand why they work and how to use them to apply them to problem solving.

So once a child knows and understands the properties of operations, what are they supposed to do with them? Isn't learning properties of operations an upper grade requirement? Why would this be important for our younger mathematicians? These are all great questions that I am frequently asked, and here are my big three reasons why they are so important:

1. They help children be flexible problem solvers. If a child comes across a multiplication problem they don't know (whether it's in isolation or in the context of a story problem), these properties give that child the opportunity to use what they know to problem solve. They can think 'could I break this problem down into smaller problems to help me solve the larger problem?' (distributive property), 'is there a different way I could group these factors to make the problem a little easier?' (associative property), or maybe even 'well I don't know my 7s facts, but I do know my 4s facts so if I switch this from being 7 x 4 to 4 x 7 then I can think about it as a 4s fact instead of a 7s fact and now I have access to solving it!' (commutative property).

2. They are the foundation of efficient symbolic strategies. If you want a child to be able to use the standard algorithm for multiplication with a solid foundation of conceptual understanding (which is what everyone who is teaching a child the standard algorithm should want), then the properties of multiplication are the gateway to that. It is critical that a child understands why the distributive property works prior to learning the standard algorithm, so that as they solve problems using the standard algorithm, they know why they can multiply one part of a factor by one part of another factor, and how to make sure they've multiplied the entire number. This 'break apart' understanding is critical to success with the standard algorithm.

3. They focus on the why, the how, and the proof, rather than just the procedure. As young mathematicians learn new skills, it is critical that they learn the why and how, not just the 'what to do' or step by step procedures for problem solving. Those are important too, but prior to learning the steps, they need to understand why they're doing what they're doing and how it works. The properties of operations really focus on that foundation behind multiplication and especially when paired with visual models, show children how multiplication works. This foundation of understanding will allow them to be much more accurate and efficient problem solver as they transition to learning multiplication with larger whole numbers (fourth grade), and eventually with decimals and fractions (fifth and sixth grade).

So what can you do to support your child/students as they learn the properties of operations? Use visual models as much as possible. These visual models are the key to helping children understand these properties and will open so many doors of understanding.

Want to learn more? Check out my podcast episodes at The Dog Ate My Homework, follow me on Instagram, and check out my engaging math resources on my Teachers Pay Teachers store!